n-strongly Gorenstein Projective and Injective and Flat Modules

YANG Xiao-yan

(Department of Mathematics,Northwest Normal University,Lanzhou 730070,China)

n-strongly Gorenstein Projective and Injective and Flat Modules

YANG Xiao-yan

(Department of Mathematics,Northwest Normal University,Lanzhou 730070,China)

In this paper,we study some properties of n-strongly Gorenstein projective, injective and f l at modules,and discuss some connections between n-strongly Gorenstein injective,projective and f l at modules.Some applications are given.

n-strongly Gorenstein projective module;n-strongly Gorenstein injective module;n-strongly Gorenstein f l at module

§1.Introduction

Unless stated otherwise,throughout this paper all rings are associative with identity and all modules are unitary modules.Let R be a ring.We denote by R-Mod(resp.,Mod-R)the category of left(resp.,right)R-modules.For any R-module M,pdRM(resp.,idRM,fdRM) denotes the projective(resp.,injective,f l at)dimension.The character module HomZ(M,Q/Z) is denoted by M+.

We assume that the reader is familiar with the Gorenstein homological dimension theory. Some references are[1-2].Nevertheless,it is convenient to give a brief history of the Gorenstein homological dimension theory.

In the sixties,Auslander and Bridger introduced the G-dimension,for f i nitely generated modules over noetherian rings[3].Several decades later,this homological dimension was extended,by Enochs et al[45],to Gorenstein projective dimension of modules that are not necessarily f i nitely generated and over not necessarily noetherian rings.And dually,they def i ned the Gorenstein injective dimension.Then,to complete the analogy with the classical homologicaldimensions,Enochs et al[6]introduced the Gorenstein f l at dimension.In the last years,the Gorenstein homological dimensions have become a vigorously active area of research(see[1] for more details).In 2004,Holm[2]generalized several results which are already obtained over noetherian rings to associative rings.Recently,Bennis and Mahdou[7]introduced a particular case of Gorenstein projective,injective and f l at modules,which are def i ned as follows.

An R-module M is called strongly Gorenstein projective(SG-projective for short)if there exists an exact sequence of projective R-modules

such that M～=Kerf and such that HomR(?,?)is exact whenever Q is a projective R-module.

The strongly Gorenstein injective(SG-injective for short)modules are def i ned dually.

An(left)R-module M is called strongly Gorenstein f l at(SG-f l at for short)if there exists an exact sequence of f l at(left)R-modules

such that M～=Kerf and such that I?RF is exact whenever I is an injective right R-module.

It is proved that the class of strongly Gorenstein projective modules is an intermediate class between the ones of projective modules and Gorenstein projective modules by[7,Proposition 2.3],which are strict by[7,Examples 2.5 and 2.13].The principal role of the strongly Gorenstein projective,injective and f l at modules is to give a simple characterization of Gorenstein projective,injective and f l at modules respectively(see[7,Theorems 2.7 and 3.5]).In 2009,Bennis and Mahdou[8]generalized the notion of strongly Gorenstein projective modules to n-strongly Gorenstein projective modules,and generalized some results of[7].Shang[9]investigated another generalization of strongly Gorenstein projective,injective and f l at modules.In current paper,we continue the study of n-strongly Gorenstein projective,injective and f l at modules, and give a detailed treatment of n-strongly Gorenstein projective,injective and f l at modules. Some applications are given.

§2.n-strongly Gorenstein Projective and Flat Modules

Def i nition 2.1[8]Let n be a positive integer.An R-module M is said to be n-strongly Gorenstein projective(n-SG-projective for short)if there exists an exact sequence

such that HomR(?,Q)leaves the sequence exact whenever Q is a projective R-module.The class of n-strongly Gorenstein projective R-modules is denoted by SGPn.An R-module M is said to be n-strongly Gorenstein injective(n-SG-injective for short)if there is an exact sequence

such that HomR(E,?)leaves the sequence exact whenever E is an injective R-module.The class of n-strongly Gorenstein injective R-modules is denoted by SGIn.Clearly,the 1-strongly Gorenstein projective modules are just the strongly Gorenstein projective modules by[7,Proposition 2.9].The 1-strongly Gorenstein injective modules are just the strongly Gorenstein injective modules.An(left)R-module M is said to be n-strongly Gorenstein f l at(n-SG-f l at for short)if there exists an exact sequence such that E?R-leaves the sequence exact whenever E is an injective right R-module.The class of n-strongly Gorenstein fl at(left)R-modules is denoted by SGFn.

Proof?Let N∈SGPn.Then there is an exact sequence

Note that 0→P1P→P→0 is exact,it follows that

is exact.Thus M～=N⊕P is n-SG-projective by[8,Proposition 2.5]and[8,Theorem 2.8].?Let M∈SGPn.Then there exists an exact sequence

Set M1=Ker(P1→M)and Mn?1=Coker(M→Pn).Then M1,Mn?1are Gorenstein projective.Consider the pushout of N⊕P→N and N⊕P→PnSince N and Mn?1are Gorenstein projective,Qnis Gorenstein projective,and so Ext1R(Qn,P)= 0.Hence Qnis projective.Consider the pullback of N→N⊕P and P1→N⊕P

Then Q1is projective.Thusexact,which implies that N is n-SG-projective by[8,Proposition 2.5]and[8,Theorem 2.8].

Corollary 2.3[10,Theorem2.1]Letbe exact with P a projective R-module.If M is SG-projective,then N is SG-projective.

ProofIf M∈SGPn,then there exists an exact sequence

Set M1=Ker(P1→M).Then we have the following exact sequences

By the middle vertical sequence and Theorem 2.2,X is n-SG-projective.Thus by the middle horizontal sequence and Theorem 2.2,N is n-SG-projective.

Corollary 2.5M is n-SG-projective if and only if there exists a short exact sequence of R-modules,where P is projective and G is n-SG-projective.

Proof?If M∈SGPn,then there exists an exact sequence

Set G=Coker(M→Pn).Then G∈SGPnandis the desired short exact sequence.?Since P,G∈SGPn,we have(M,Q)=0 for all i≥1 and any projective R-module Q.On the other hand,since G∈SGPn,there exists an exact sequencewith each Piprojective.Set G1=Ker(P1→G). Consider the exact sequencesThen G1⊕P～=M⊕P1.Since

is exact,it follows from the proof of Theorem 2.2 that

Let X be a class of R-modules.We call X projectively resolving[2]if(1)it contains all projective R-modules;(2)for every short exact sequence 0→X′→X→X′′→0 with X′∈X the conditions X′∈X and X∈X are equivalent.An injectively resolving class is def i ned dually.

Theorem 2.6The following are equivalent

(1)The class SGPnis closed under extensions;

(2)The class SGPnis projectively resolving;

(3)All Gorenstein projective R-modules are n-SG-projective;

(4)For every short exact sequencewith G0,G1∈SGPn.If Ext1R(M,Q)=0 for any projective R-module Q,then M is n-SG-projective.

Proof(1)?(2)To claim that the class SGPnis projectively resolving,it suffices to prove that it is closed under kernels of epimorphisms.Letbe exact with B,C∈SGPn.We prove that A is n-SG-projective.Since C is n-SG-projective,there exists an exact sequencewith each Piprojective. Set C1=Ker(P1→C).Consider the pullback of B→C and P1→C

By Corollary 2.4,C1is n-SG-projective and so D is n-SG-projective by(1).Thus A is n-SG-projective by Theorem 2.2.

(2)?(3)Let M be a Gorenstein projective R-module.Then there is a Gorenstein projective R-module N such that M⊕N is SG-projective by[7,Theorem 2.7].Set L=M⊕N⊕M⊕N⊕···. Then L is SG-projective and so L is n-SG-projective by[8,Proposition 2.5].Consider the following exact sequence

(3)?(4)It follows from[2,Corollary 2.11].

By analogy with the proofs of theorems 2.2 and 2.6,we have the following dual results.

Theorem 2.8The following are equivalent

(1)The class SGInis closed under extensions;

(2)The class SGInis injectively resolving;

(3)All Gorenstein injective R-modules are n-SG-injective;

(4)For every short exact sequencewith G0,G1∈SGIn.If Ext1R(E,M)=0 for any injective R-module E,then M is n-SG-injective.

Proposition 2.9If M is an n-SG- fl at left R-module,then M+is an n-SG-injective right R-module.

ProofLet M∈SGFn.Then there exists an exact sequence

Proposition 2.10Let R be two-sided coherent with FP-id(RR)＜∞.If M is an n-SG-injective right R-module,then M+is n-SG-f l at.

ProofLet M∈SGIn.Then there exists an exact sequence

A ring R is said to be n-FC if R is two-sided coherent with FP-id(RR)≤n,FP-id(RR)≤n. A ring R is said to be n-Gorenstein if R is two-sided noetherian with id(RR)≤n,id(RR)≤n.

Corollary 2.11Let R be an n-FC ring.If M is an n-SG-injective right R-module,then M+is an n-SG- fl at left R-module.

Corollary 2.12Let R be an n-Gorenstein ring.Then M is an n-SG-injective right R-module,then M+is an n-SG- fl at left R-module.

Proposition 2.13Let R be left coherent with FP-id(RR)＜∞.Then every n-SG-projective left R-module is n-SG- fl at.

ProofLet M∈SGPn.Then there is an exact sequence of left R-modules

such that HomR(?,Q)leaves the sequence exact whenever Q is a projective left R-module.Let I be an injective right R-module.Then fdRI＜∞by assumption.We use induction can to show that

is exact,which implies that M is n-SG-f l at.

A ring R is said to be left(resp.,right)m-perfect if every f l at left(resp.,right)R-module has projective dimension less than or equal to m.

Proposition 2.14Let R be right coherent left m-perfect.Then every n-SG-projective left R-module is n-SG-f l at.The converse holds when m=0.

ProofLet M∈SGPn.Then there is an exact sequence of left R-modules

such that HomR(?,Q)leaves the sequence exact whenever Q is a projective left R-module.Let I be an injective right R-module.Then pdRI+≤m by assumption.So we have the following commutative diagram

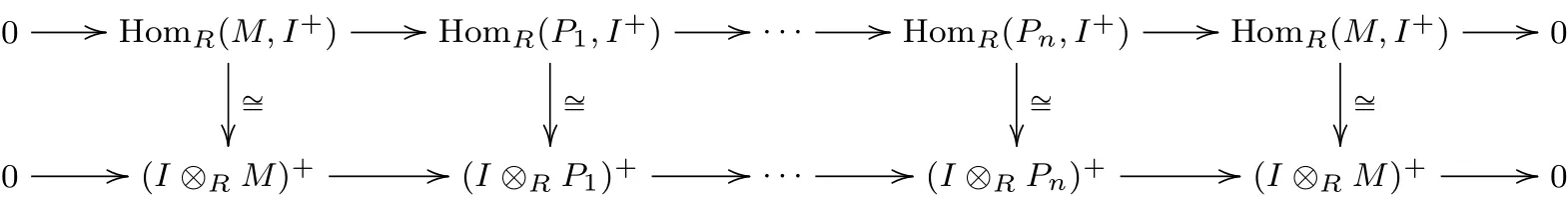
Conversely,let M∈SGFn.Then there is an exact sequence of left R-modules

such that I?R?leaves the sequence(?)exact whenever I is an injective right R-module.Let P be a projective left R-module.Thenis split by assumption.But HomR(?,P++)～=(P+?R?)+and P+is injective,so P+?R?leaves the sequence (?)exact,and hence HomR(?,P++)leaves the sequence(?)exact.This implies that HomR(?,P) leaves the sequence(?)exact since P is isomorphic to a summand of P++.Therefore M is n-SG-projective.

§3.Excellent Extension

In this section,we give some applications of n-strongly Gorenstein injective,projective and fl at modules.We begin with the following de fi nition.

(1)The ring S is called right R-projective in case for any right S-module MSwith an S-submodule NS,NR|MRimplies NS|MS.For example,every n×n matrix ring Mn(R)is right R-projective.

(2)The ring extension S≥R is called a fi nite normalizing extension in case there is a fi nite subset{s1,···,sn}of S such that

(3)A f i nite normalizing extension S≥R is called an excellent extension in case condition (1)is satisf i ed andRS,SRare free modules with a common basis{s1,···,sn}.Excellent extensions were introduced by Passman[11].Example include n×n matrix rings,and crossed products R?G where G is a f i nite group with|G|?1∈R.

Proposition 3.1Assume that S≥R is an excellent extension.Then

(a)RM∈SGPn(R)if and only if S?RM∈SGPn(S)for all M∈R-Mod;

(b)RM∈SGIn(R)if and only if HomR(S,M)∈SGIn(S)for all M∈R-Mod;

(c)MR∈SGFn(R)if and only if M?RS∈SGFn(S)for all M∈Mod-R.

Proof(a)?Let M∈SGPn(R).Then there exists an exact sequence

in R-Mod with every Piprojective.So

is exact in S-Mod with each S?RPiprojective.LetQˉ be any projective left S-module.Then Qˉ is a projective left R-module,and so ExtiS(S?RM,Qˉ)～=ExtiR(M,Qˉ)=0 for all i≥1.It follows that S?RM∈SGPn(S).

?By assumption,there exists an exact sequence

in S-Mod with eachˉPiprojective.For a projective left S-moduleˉP,there is a projective left S-moduleˉP′such thatˉP⊕ˉP′=S?RˉP.By analogy with the proof of[10,Proposition 3.10], we obtain an exact sequence

in S-Mod with eachˉQiandˉQ projective.Thenis exact in R-Mod with eachQˉiandQˉ projective.Let Q be any projective left R-module.Then S?RQ is a projective left S-module.Thus 0=(S?RM,S?RQ)～=(M,S?RQ), and so(M,Q)=0 for all i≥1 since Q is isomorphic to a summand of S?RQ.It follows that M∈SGPn(R).

(b)?Let M∈SGIn(R).Then there exists an exact sequence

in R-Mod with every Eiinjective.Then

is exact in S-Mod with every HomR(S,Ei)injective.LetIˉbe any injective left S-module.Then Iˉis an injective left R-module and so ExtiS(Iˉ,HomR(S,M))～=ExtiR(Iˉ,M)=0 for all i≥1. Hence HomR(S,M)∈SGIn(S).

and so ExtiR(I,M)=0 for all i≥1 since I is isomorphic to a summand of HomR(S,I).Hence M∈SGI(R).

(c)?Let M∈SGFn(R).Then there exists an exact sequence

in Mod-R with every Fif l at.So

is exact in Mod-S with each Fi?RS fl at.LetIˉbe any injective left S-module.LetIˉbe any injective left S-module and let F be a fl at resolution ofIˉ.Then TorSi(M?RS,Iˉ)= Hi((M?RS)?SF)～=Hi(M?RF)=TorRi(M,Iˉ)=0 for all i≥1 and so M?RS∈SGFn(S).

?By assumption,there extsts an exact sequence

in Mod-S with eachˉFif l at.For a f l at right S-moduleˉF,there is a f l at right S-moduleˉF′such thatˉF⊕ˉF′=ˉF?RS.By analogy with the proof of[10,Proposition 3.10],we obtain the following exact sequence

in Mod-S with eachˉFiandˉF f l at.Then is exact in Mod-R with eachFˉiandFˉ fl at.Let I be any injective left R-module.Then HomR(S,I)is an injective left S-module.Let F be a fl at resolution of M over R.Then 0=TorSi(M?RS,HomR(S,I))=Hi((F?RS)?SHomR(S,I))～=Hi(F?RHomR(S,I))= TorRi(M,HomR(S,I))for all i≥1 and so TorRi(M,I)=0.Hence M∈SGFn(R).

Corollary 3.2Let R?G be a crossed product,where G is a f i nite group with|G|?1∈R.

(a)For any M∈(R?G)-Mod,RM is n-SG-projective if and only if(R?G)?RM is n-SG-projective;

(b)For any M∈(R?G)-Mod,RM is n-SG-injective if and only if HomR(R?G,M)is n-SG-injective;

(c)For any M∈Mod-(R?G),MRis n-SG-f l at if and only if M?R(R?G)is n-SG-f l at.

Corollary 3.3Let R be a ring m any positive integer.Then

(a)For any M∈Mm(R)-Mod,RM is n-SG-projective if and only if Mm(R)?RM is n-SG-projective;

(b)For any M∈Mm(R)-Mod,RM is n-SG-injective if and only if HonR(Mm(R),M)is n-SG-injective;

(c)For any M∈Mod-Mm(R),MRis n-SG-f l at if and only if M?RMm(R)is n-SG-f l at.

Corollary 3.4Assume that S≥R is an excellent extension.Then

(a)RM∈SGP(R)if and only if S?RM∈SGP(S)for all M∈R-Mod;

(b)RM∈SGI(R)if and only if HomR(S,M)∈SGI(S)for all M∈R-Mod;

(c)MR∈SGF(R)if and only if M?RS∈SGF(S)for all M∈Mod-R.

Corollary 3.5Assume that S≥R is an excellent extension.Then

(a)RM∈GP(R)if and only if S?RM∈GP(S)for all M∈S-Mod;

(b)RM∈GI(R)if and only if HomR(S,M)∈GI(S)for all M∈S-Mod;

(c)MR∈GF(R)if and only if M?RS∈GF(S)for all M∈Mod-S.

Proof(a)?Let M∈GP(R).There is an N∈SGP(R)such that M is a direct summand of N.By Corollary 3.4,S?RN∈SGP(S).So S?RM∈GP(S).

?Since S?RM∈GP(S)andSM is isomorphic to a summand ofS(S?RM),we see thatSM∈GP(S).Then there exists a projective resolution of left S-modules

with M～=Im(ˉP0→ˉP0),such that HomS(ˉ?,ˉ?)is exact for any projective left S-modulesˉQ. Let Q be any projective left R-module.Then HomR(S,Q)|Qtfor some t∈?.Thus HomR(S,Q) is a projective left R-module and HomR(S,Q)is a projective left S-module,which implies that

is exact.SinceˉPi,ˉPiare projective left R-modules for every i,we haveRM∈GP(R).

(b)and(c)It is by analogy with the proof of(a).

[1]ENOCHS E E,JENDA O M G.Relative Homological Algebra[M].Berlin:de Gruyter Exp Math Vol 30, Walter de Gruyter and Co,2000.

[2]HOLM H.Gorenstein homological dimensions[J].J Pure Appl Algebra,2004,189(1-3):167-193.

[3]AUSLANDER M,BRIDGER M.Stable Module Theory[M].Mem Amer Math Soc:Providence R I,1969.

[4]ENOCHS E E,JENDA O M G.On Gorenstein injective modules[J].Comm Algebra,1993,21(10):3489-3501.

[5]ENOCHS E E,JENDA O M G.Gorenstein injective and projective modules[J].Math Z,1995,220(1): 611-633.

[6]ENOCHS E E,JENDA O M G,TORRECILLAS B.Gorenstein f l at modules[J].Nanjing Daxue Xuebao(Shuxueban Niankan),1993,10(1):1-9.

[7]BENNIS D,MAHDOU N.Strongly Gorenstein projective,injective and f l at modules[J].J Pure Appl Algebra, 2007,210(2):437-445.

[8]BENNIS D,MAHDOU N.A generalization of strongly Gorenstein projective modules[J].J Algebra and Its Applications,2009,8(2):219-227.

[9]SHANG Wen-liang.Notes on strongly n-Gorenstein projective,injective and f l at modules[J].Chin Quart J Math,2012,27(3):2012 389-396.

[10]YANG Xiao-yan,LIU Zhong-kui.Strongly Gorenstein projective,injective and f l at modules[J].J Algebra, 2008,320(7):2659-2674.

[11]PASSMAN D S.The Algebraic Structure of Group Rings[M].New York:Wiley-Interscience,1977.

tion:18G25,16E30

1002–0462(2014)04–0553–12

date:2013-02-25

Supported by the National Natural Science Foundation of China(11361051);Supported by the Program for New Century Excellent the Talents in University(NCET-13-0957)

Biography:YANG Xiao-yan(1980-),female,native of Zhangye,Gansu,an associate professor of Northwest Normal University,Ph.D.,engages in homological algebra.

CLC number:O153.3Document code:A